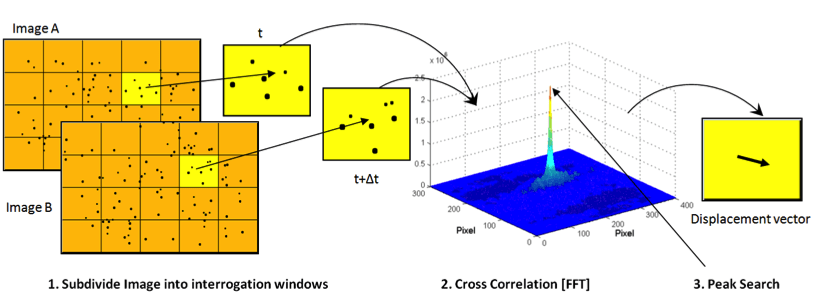
DIC is a classic pattern recognition technique, which is used in PIV, surface displacement analysis (SDA), particle tracking, speckle velocimetry, and other experimental techniques. DIC provides sufficient resolution for most practical geotechnical applications. It compares two images in order to determine the magnitude and direction of displacement. The standard correlation function, C, is given by:
C (Δx,Δy) = ∫∫ Io (x , y) I1 (x+Δx , y+Δy) dx dy
(1) Where x and y are dimensions in the interrogating area A, and I0 and I1 are the gray-scale intensities of the 2 images being compared (image 0 and 1). The correlation function is applied repeatedly while shifting the images by distances Δx andΔy. When two images undergo a displacement, the location of the peak of the correlation function determines the location of the best match between the two images, which corresponds to the magnitude and direction of the movement.
The key steps of DIC are illustrated above, where the gray scale intensities of two images are correlated using Eq. 1. The peak of the cross-correlation function represents the comparative movement between the two images. Successive displacement can be found by correlating successive images and locating the peaks. If the whole image area is divided into many small interrogation windows, the whole displacement field can be obtained by calculating the displacement in each interrogation window. An advanced form of DIC which utilizes variable window sizing, window shifting as shown below.



A Fast Fourier Transform (FFT) is often utilized to speed up calculations, but its application is not essential for DIC.The technique has been adapted for digital image analyses of transparent soils with an accuracy on the order of ±1%.
DIC analysis typically results in incremental displacements of interrogation windows. It is often desirable to calculate the cumulative displacements resulting from soil movements. To do so, incremental displacements may be added at fixed DIC interrogation window center points over a sequence of analyses. This results in cumulative displacements in a Eulerian framework. However, in some problems, it is expected that regions with large field gradients can occur. In such cases, integration of incremental displacements from fixed DIC nodal points can result in significant error. It is, therefore, desirable to obtain Lagrangian displacements for such high-gradient problems. To do so, a method where incremental displacements are used to update the DIC mesh, by means of Delaunay triangulation and linear interpolation. The method allows for probing large displacements, with high field gradients.
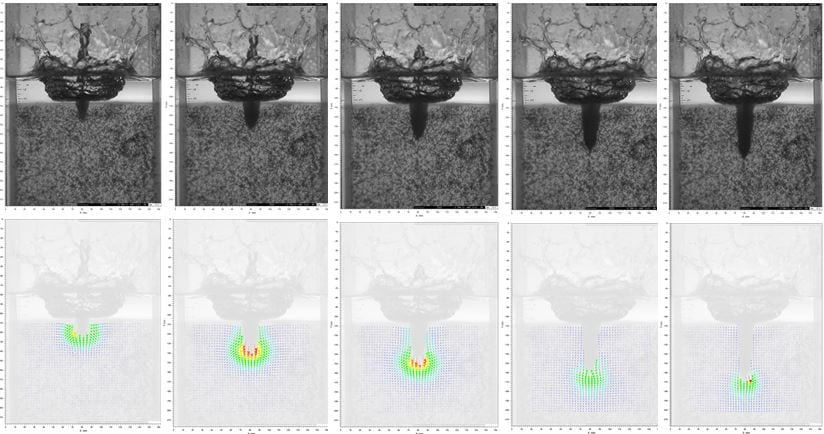
The following images and video depict DIC of a projectile penetrating in transparent soils. For further details see the Ballistics tab under applications.
For more information about multi-scale analysis for granular image correlation please visit MagicGeo
Primary References
- Chen, Z., K. Li, M. Omidvar, M. Iskander (2017), Guidelines for DIC in geotechnical engineering research,” J. of Physical Modelling in Geotechnics, Vol.17, No.1, pp. 3–22, doi: 10.1680/jphmg.15.00040, ICE [link]
- Omidvar, M., Z. Chen, and M. Iskander (2014), Image-based lagrangian analysis of granular kinematics. Journal of Computing in Civil Engineering. Vol. 29, No. 6, doi: 10.1061/cp.1943-5487.0000433, ASCE [link]
- Sadek, S M. Iskander & J. Liu (2005), Closure to accuracy of digital image correlation for measuring deformation in transparent media. Journal of Computing in Civil Engineering. Vol. 19, No. 2, pp. 219-222, ASCE [link]
- Liu, J. and M. Iskander (2004), Adaptive cross correlation for imaging displacements in soils. Journal of Computing in Civil Engineering, Vol. 18, No. 1, pp. 46–57, ASCE [link]
- Sadek, S., M. Iskander, and J. Liu (2003). Accuracy of digital image correlation for measuring deformations in transparent media. Journal of Computing in Civil Engineering. Vol. 17, No. 2, pp. 88-96, ASCE [link]