Update (15/2/2018): the paper now has a DOI number! https://doi.org/10.1016/j.jcp.2018.01.031
Numerical models of the ocean (and of the atmosphere) are always underresolved. In the ocean tracers such as temperature, salinity, dissolved oxigen, iron and other nutrients have been measured to have fluctuations down to tiny scales, which in some instances may be submillimetric. Even a regional model that only encompasses a limited portion of the world’s oceans (say, just the Gulf) can only resolve, in the best of cases, scales of the order of one kilometer (and often much less).
Just about any existing ocean model works by representing the relevant physical quantities on a fixed grid, spanning latitude, longitude and depth. This is called an Eulerian representation. What happens when some structure (say, fluctuations in temperature) becomes so small as to be unresolvable on a model’s grid? Details change from model to model, but, by and large, all model smear out the fluctuations, so that the values recorded at the grid nodes are representative not of the pointwise value of that quantity, but of its average over a region of size comparable with the size of a computational mesh.
That’s the same thing that happens when you look at a newspaper’s page from a distance too large to discern the individual characters that compose the printed words. In that case you don’t see black letters printed over white paper: you see gray areas corresponding to the region of the page covered by text.
Now imagine that there were bugs living by eating concentrated ink: on a real newspaper page those bugs would thrive, at least until they hadn’t eaten every printed word. But if you attempted to model that process with an unresolved model, it would not quite work in the same way: because the simulated bugs would only be offered a smeared-out version of reality, they might find that the ink is not concentrated enough to survive. And thus the model may wrongly predict that the bugs would get extinct without wiping out all the ink from the page.
Biases of this sort are commonplace in ocean models that attempt to go beyond physics, and describe the chemical and biological processes that occur in seawater. What can be done to fix that problem?
At first sight, the situation appears hopeless, because there’s no way that a computer will have enough number crunching strength to reach the necessary resolution in the foreseeable future. But not all is lost. Lack of resolution is bad in itself, but it becomes truly evil when it smears out the fluctuations that would otherwise be present. Is it then possible to perform simulations that, albeit underresolved, still retain a realistic range of fluctuations at the smallest resolved scales? If the answer were “yes” then we could avoid the worst of the problems that comes with underresolution.
Well, it turns out that the answer is “yes”. But the price to pay is to change completely the simulation framework. Rather than representing quantities on a fixed grid, we must think in terms of moving water masses, going with the flow, each carrying a certain amount of temperature, salinity, oxygen, etc. This is called a Lagrangian description of the flow, and is immune from the smearing effects that are inevitable with the Eulerian approach.
My last paper, just accepted for publication on Journal of Computational Physics, works out the gory mathematical details and describes a general framework for simulation biogeochemical processes without incurring in nasty biases due to the smearing-out effect.
As an example of the performance of the method, here are two simulations. The one on the left is obtained with a high-resolution code (\(4096^2\) grid nodes). On the right, the same simulation is performed with the Lagrangian approach, using just \(128^2\) particles.
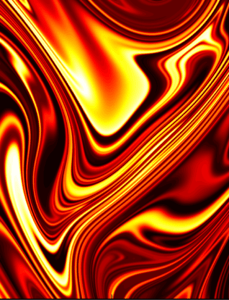

Even though the Lagrangian simulation is underresolved, and (by design) can’t capture all the delicate intricacies of the orange filaments visible in the high-resolution simulation, the range of fluctuations, and the overall shape of the structures is the same in both simulations. This is what really matters for ocean biogeochemistry: we want to get the statistics right! And with a Lagrangian approach we will…