Abstract.
The Doppler formula (1)
$f=f_0 ({1 \pm \frac{v { \ }cos \theta}{c}})$ (1)
is not correct for electromagnetic waves as well as for acoustic waves with spherical or circular wavefronts.
In the case of electromagnetic waves or acoustic waves with spherical or circular wavefronts, the correct result can be obtained using formula (2):
$f = f_0 \cdot ( \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})$ (2).
In the last formula, with the letter $\delta$ we marked the angle between the vectors $v$ and $c$.
1. Introduction.
For this project, we accept the same conditions that are usually accepted for the Doppler Effect.
There are various parameters that can be used to describe the waves. Waves can be linear or non-linear. The waveform can be sinusoidal, square, etc. The waves can be transverse or longitudinal. An electromagnetic wave can have different polarization. These parameters are not very important for Doppler theory.
Important parameters for the Doppler Effect are: wave frequency, wave coherence, wavefront shape, and also the speed and direction of wave propagation.
In this project, we pay special attention to the wavefront shape. The wavefront shape, whether it is a point, line or surface, is defined as:
$D=c \cdot t$ (3).
Here D is the distance the wave has traveled from its source in time t.
A wavefront is a set of points with the same phase of the wave. It can be a single point, line or surface. If the wave starts from the source at time zero, then the wave front is a set of points located at the maximum distance from the wave source.
The wave front can be associated with points of wave maximum, wave minimum, or any other set of points with the same phase, which leave the wave source at the time of the start of the experiment. A linear wavefront should not be confused with the general definition of a linear wave. The electromagnetic wave is linear in the sense of equation (4):
$ \frac{\partial^2 u}{\partial x^2} + \frac{\partial^2 u}{\partial y^2} + \frac{\partial^2 u}{\partial z^2} =\frac{1}{c^2} \frac{\partial^2 u}{\partial t^2}$ (4).
Functions (5) represent the solution of equation (4) for an electromagnetic wave:
$u=A \cdot e^{i \omega t}$, $r=c \cdot t$ (5).
At the same time the wavefront of electromagnetic wave has spherical shape. The spherical symmetry of the wavefront of an electromagnetic wave follows from the symmetry of the Maxwell-Hertz equation (6):
$ \left \{ \begin {array} {lll}\frac{1}{c} \frac{\partial E _x}{\partial t} =\frac{\partial H _z}{\partial y} – \frac{\partial H _y}{\partial z} \\ \frac{1}{c} \frac{\partial E _y}{\partial t} =\frac{\partial H _x}{\partial z} – \frac{\partial H _z}{\partial x}\\ \frac{1}{c} \frac{\partial E _z}{\partial t} =\frac{\partial H _y}{\partial x} – \frac{\partial H _x}{\partial y} \end {array} \right \} $ $ \left \{ \begin {array} {lll}-\frac{1}{c} \frac{\partial H _x}{\partial t} =\frac{\partial E _z}{\partial y} – \frac{\partial E _y}{\partial z} \\ -\frac{1}{c} \frac{\partial H _y}{\partial t} =\frac{\partial E _x}{\partial z} – \frac{\partial E _z}{\partial x}\\ -\frac{1}{c} \frac{\partial H _z}{\partial t} =\frac{\partial E _y}{\partial x} – \frac{\partial E _x}{\partial y} \end {array} \right \} $ (6).
The spherical shape of the wavefront of electromagnetic wave is also explicitly expressed in the Minkowski’s equation:
$x^2+y^2+z^2=c^2t^2$ (7).
Equation (7) describes the propagation of the wavefront of an electromagnetic wave as a sphere with a radius . The purpose of this introduction is to prevent confusion when describing the wavefront shape. Despite the fact that the electromagnetic wave is linear, transverse and has a sinusoidal shape, we will characterize it in this project as a wave with a spherical wave front according to equation (7). The linear or spherical wave front should also not be confused with linear or circular polarization of the wave.
A similar explanation applies to acoustic spherical or circular waves, such as waves created by raindrops on the surface of the water. These waves can be considered as linear waves in accordance with the equation:
$ \frac{\partial^2 u}{\partial x^2} + \frac{\partial^2 u}{\partial y^2} =\frac{1}{c^2} \frac{\partial^2 u}{\partial t^2}$ (8).
But in this project, it is more important for us to consider them as waves with a circular wavefront according to the equation:
$x^2+y^2=c^2 \cdot t^2$
$u=A \cdot cos(\omega t)$, $r=c \cdot t$ (9).
2. Theory of Doppler. Historical view.
Christian Doppler noticed changes in the observed wave frequency when the boat crossed the waves in the direction of wave movement and in the opposite direction. The difference in the observed wave frequency is called the Doppler Effect. In 1842, Doppler [1] introduced formula (10) for the case when the vectors v (detector velocity) and c (wave velocity) are collinear:
$f=f_0 ({1 \pm \frac{v }{c}})$ (10).
In this formula, $f$– is the observed or measured frequency in moving coordinates, $f_0$- is the initial frequency measured at the wave source in coordinates at rest. If the wave source is not at rest, then instead of the speed of the detector, we must take the difference between the speed of the wave source and the speed of the detector. Below we assume that the wave source is at rest.
Eventually, the Doppler formula (10) was extended to the case of non-collinear vectors $v$ and $c$:
$f=f_0 ({1 \pm \frac{v { \ }cos \theta}{c}})$ (11).
Below we analyze the logic of Doppler experiments and the validity of formula (11) for waves with different wavefront shapes.
3. The experiment.
Our initial experiments with one-dimensional waves, which were performed with wave generators and recording systems, were subsequently complemented by computer simulation of experiments.
In computer simulations, linear or circular waves were created using Blender software.
The main result of our experiments is not numerical parameters or graphs, but our experimental method itself, which allowed us to correct the Doppler formula and present the results in the form of new formulas both for waves with a linear wavefront and for waves with a spherical or circular wavefront for which the Doppler formulas are incorrect.
4. The case of collinear vectors $v$ and $c$.
At the beginning of this project we made a statement that Doppler formula (1) is not correct for spherical, circular and non-orthogonal linear waves. It means that we cannot use Doppler formula as an argument in our analysis. We have to prove the validity of Doppler formula itself, using the very basic definitions of wave frequency, wave period and wavelength.
We begin by analyzing a wave that changes amplitude in the direction of the vertical axis and propagates along the horizontal axis.
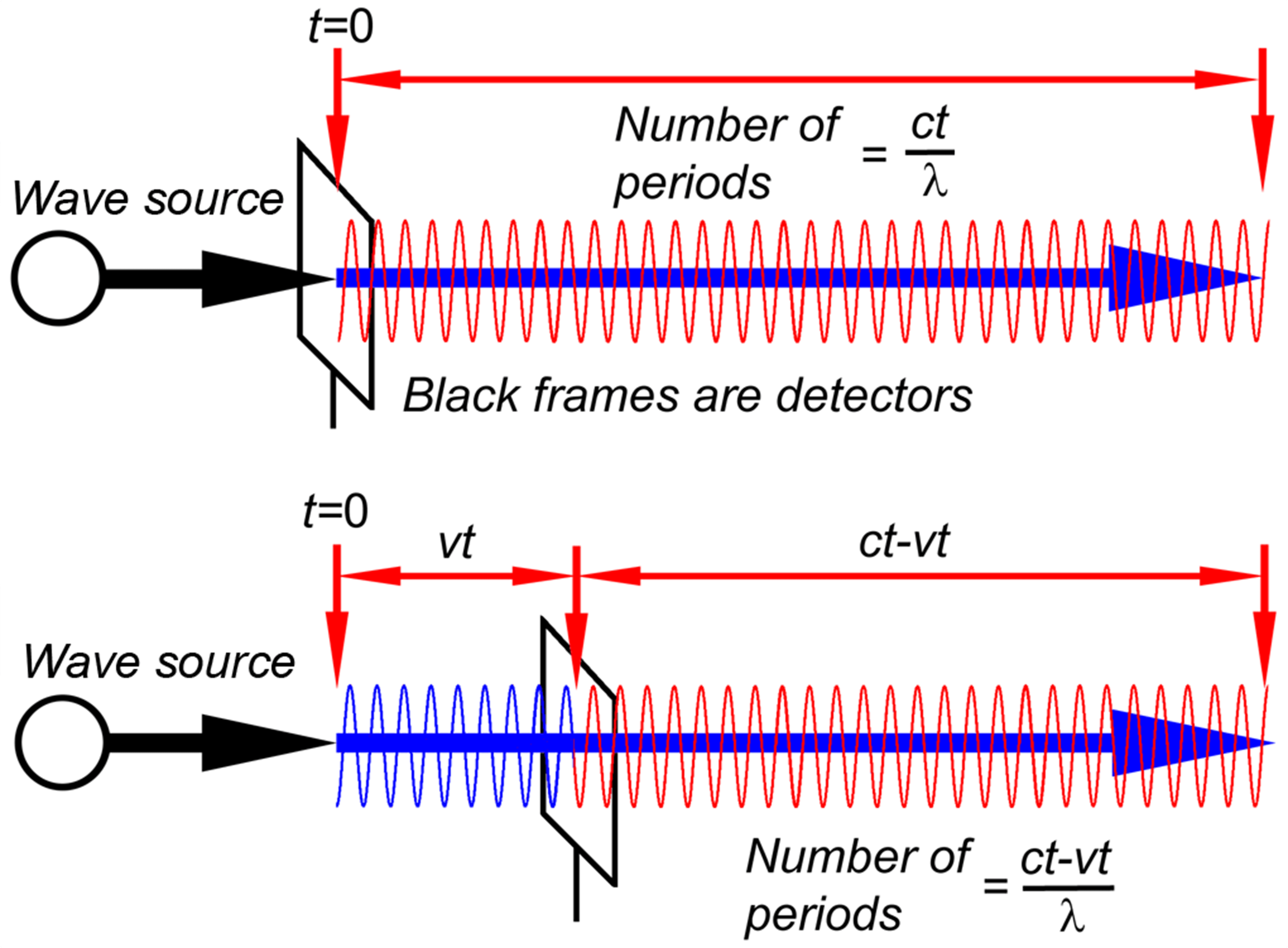
Fig. 1. Collinear Doppler Effect.
Fig. 1 shows the case of collinear motion of the wave and detector, which means that the vectors v and c move along one straight line. We have chosen the initial time so that the position of the wave front coincides with the wave maximum.
The black frames in Fig. 1 show the position of the detectors. Each time a wave maximum passes through the detector, we count one wave period or one wavelength.
When the wave source and detector are at rest, the wave travels the distance:
$D=c \cdot t$ (12).
The number of wave maxima passing through the detector during time $t$ is equal to:
$k=\frac{c \cdot t}{\lambda}$ (13).
If the time interval is equal to 1 second, then the number of periods or wave oscillations per second is called the wave frequency:
$f=k=\frac{c}{\lambda}$ (14).
When the detector moves at a speed $v$ collinear with the direction of wave propagation, and its direction of movement toward the light source, the number of wave periods that the detector calculates will be equal to:
$k=\frac{c \cdot t + v \cdot t}{\lambda}$ (15).
In equation (15), the letter $k$ denotes the number of wave maxima or the number of wave periods.
When the wave and detector move collinearly in the same direction, the number of wave periods is equal to:
$k=\frac{c \cdot t – v \cdot t}{\lambda}$ (16).
If the time interval is equal to 1 second, then $k$ is equal to the wave frequency:
$f=\frac{c \pm v}{\lambda} = \frac{c \pm v}{c} \cdot \frac{c}{\lambda} = f_0 \cdot (1 \pm \frac{v}{c})$ (17).
Equation (17) is the Doppler formula for collinear vectors $v$ and $c$.
Thus, we confirmed that for a one-dimensional wave, in the case of collinear vectors $v$ and $c$, the Doppler formula (17) and our experiment give the same correct result.
Now we can use this formula as a reference for analysis of other types of waves.
5. The case of the non-collinear vectors $v$ and $c$.
This is the case, when the wave propagates along the surface.
For non – collinear vectors $v$ and $c$ equation (17) is usually replaced by equation (18):
$f= f_0 \cdot (1 \pm \frac{v \cdot cos \theta}{c})$ (18).
The reason for replacing the velocity $v$ in formula (17) with the expression ($v \cdot cos \theta$) in formula (18) requires explanation. Sometimes such a replacement is justified by the phrase: “because ($v \cdot cos \theta$) is the projection of the vector $v$ onto the direction of the vector $c$”. But still, it is necessary to explain the link between projection and frequency. Below we analyze the accuracy of this statement and indicate the limits of applicability of formula (18).
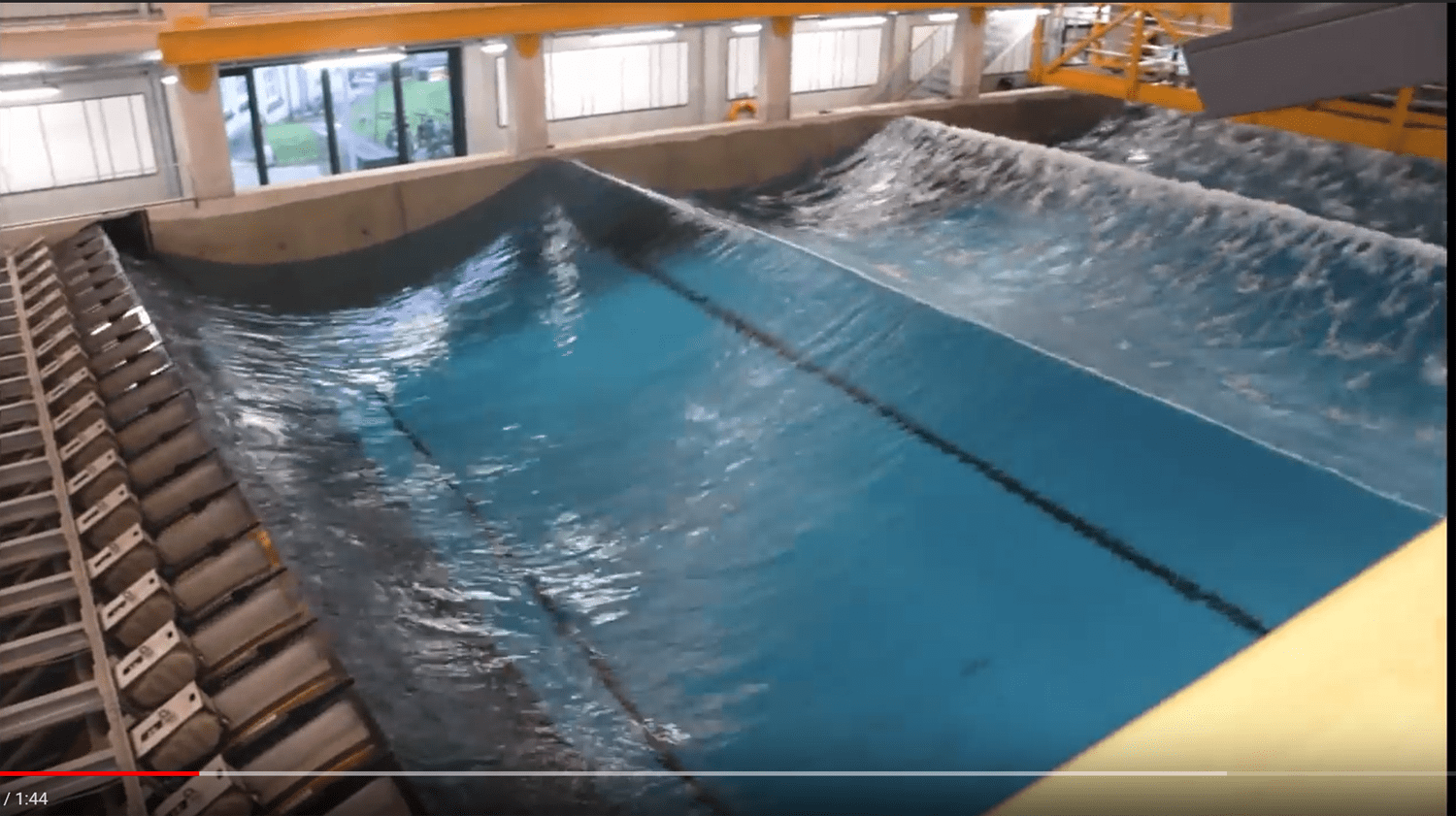
Fig. 2. The waves with linear orthogonal wavefront. Plymouth university hydrodynamics facility. Image from YouTube.
Fig. 2 shows a wave with a linear orthogonal wavefront. The positions of the maxima of this wave in the direction perpendicular to the wave propagation vector are represented by a straight line. A wave of this type has a linear front that is orthogonal to the wave propagation direction vector.
In our experiment, we check the positions of the wave maxima. One of these lines can be selected as the wavefront line.
We would like to note that it is possible to divide the space between two maxima into an infinite number of parallel lines. Each line will represent many points with the same phase of the wave. Our experimental method can be applied to any such line. Each of these lines is parallel to the wave front. Each can be selected as a wavefront. This approach guarantees an accurate result.
6. The case of a wave with a linear orthogonal wavefront.
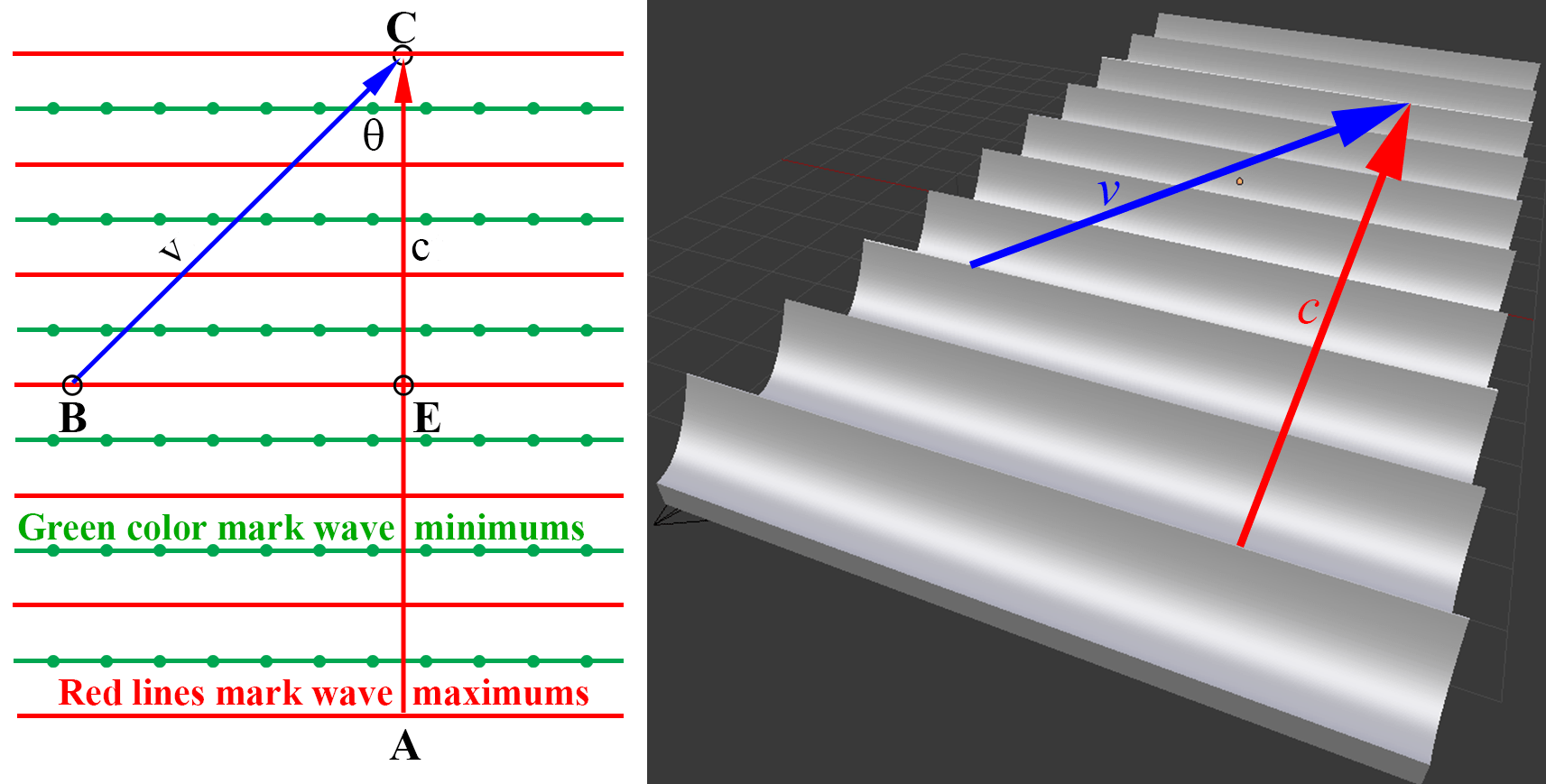
Fig. 3 (right). Isometric view of a waves with a linear orthogonal wavefront.
Fig. 4 (left). Top view on the waves with linear orthogonal wavefront.
One detector on Fig. 4 is located at point B and the other at point E above the wave. Each detector counts the number of wave maxima that passed under their respective positions. Solid red lines in Fig. 4 mark the positions of the wave maxima. Dotted green lines indicate the positions of the wave minima.
In our experiment, at the initial time, the detectors are located at points B and E. We must be sure that our detectors move along the BC and EC paths, respectively. We also need to make sure that the line BE, which connects the positions of our detectors, is perpendicular to the direction of propagation of the wave EC at any point in time during our experiment.
To ensure these conditions, we prepared two thin cover plates with slots. These two plates are shown in Fig. 5 and Fig. 6. The slots in the plates are marked with black lines around arrows.
The first plate in Fig. 5 has two slots, one above the line BC and the other above the line EC. This first plate is fixed relative to the wave source. The first detector, which begins to move from point B and is connected to this slot, will move along line BC.
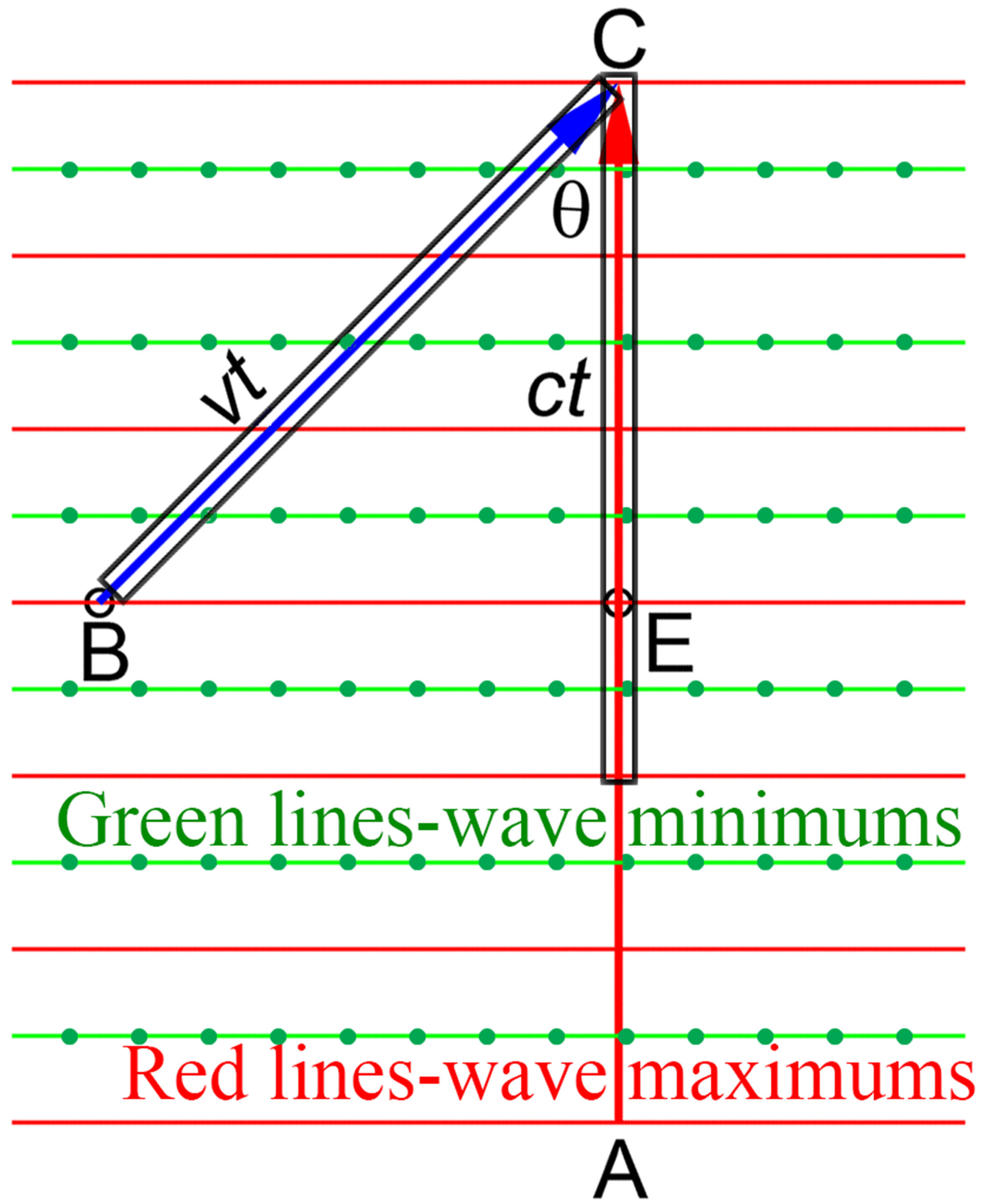
Fig. 5. Position of the slits in the lower cover plate 1.
The second plate can be moved along rails parallel to the EC line. These rails allow the second plate to move parallel to the direction of wave propagation. The second plate is located above the first plate and has a slot along the BE line, which is parallel to wavefront (Fig. 6). While both detectors are not moving, we note that each subsequent wave maximum is recorded simultaneously by both detectors at positions B and E.
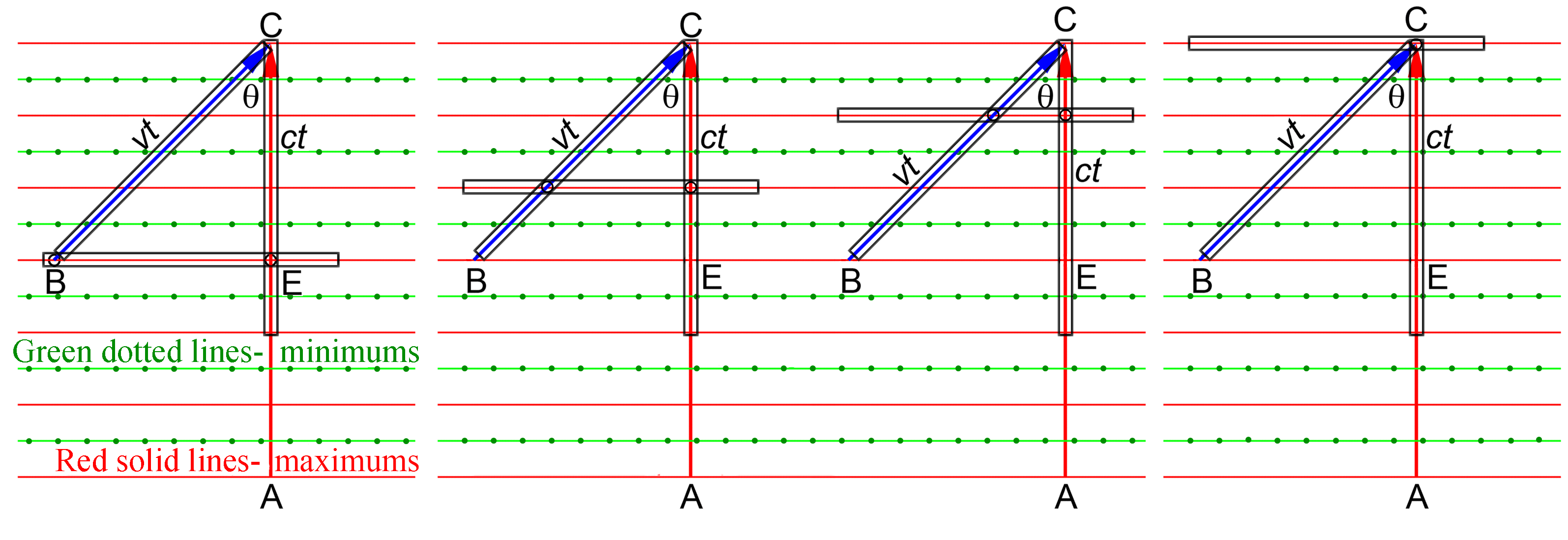
Fig. 6. Position of the slit in the upper plate during experiment.
In this geometry of the experiment, we move the top plate on the guides in the direction of the AC line. At the same time, our detectors connected to both planes will move along the lines BC and EC, as shown in Fig. 6. The transit time for the distances BC and EC is the same for both detectors. Both detectors start and end motion simultaneously.
7. The result of experiments with waves, which have a linear orthogonal wavefront.
First, it should be noted that from the beginning to the end of our experiment, each wave maximum was detected simultaneously by detector B and detector E. The same result was observed both when the detectors did not move and when both detectors moved. The result remained unchanged for different speeds of detectors and waves.
The phase of the wave measured by both detectors was the same at any time during our experiment. The result of measuring both a collinear and non-collinear detector is the same wave frequency. This experimental result can be expressed as:
${Sp}_E = {Sp}_B \cdot cos { \theta} $ (19).
Taking into account that (${Sp}_E$) is the velocity of the collinear detector, and (${Sp}_B$) is the velocity of the non-collinear detector, we experimentally proved that replacing $v$ in formula (17) with (${v \cdot cos \theta}$) in formula (18) for non-collinear velocities $v$ and $c$ gives the correct result.
This experiment confirmed that for waves with linear orthogonal wavefronts, in which the line of wavefront is parallel to the BE line in Fig. 6, the Doppler formula (17) is correct.
This experiment also demonstrated that for waves with a spherical wavefront, as well as for other waves with a wavefront that is not perpendicular to the direction of wave propagation, the Doppler formula is incorrect, because the line of wavefront will not coincide with the line of projection.
The Doppler formula cannot be used for electromagnetic waves. Arguments about the projection of the velocity vector, as well as attempts to average or integrate the angles along the circular wave front, are incorrect.
8. Waves with a linear non – orthogonal wave fronts.
This type of waves is not very popular in theoretical physics. In ideal media linear waves generated by a flat surface propagate in the direction, which is orthogonal to the wavefront.
However, in the nature, waves with linear wavefront, which is not orthogonal to the direction of wave propagation can be observed on the water surface as a result of action of two or more parameters. Waves with linear orthogonal wavefront, created by the river current are transformed into waves with non-orthogonal wavefront under the action of wind, which blows across the river. Ocean waves coming onshore become waves with non-orthogonal wavefront as a result of interaction with rip current. Wave’s interference or change of the water depth can also create waves with not-orthogonal wavefront.
We need to analyze experiment with this type of waves in order to further develop our method, as well as for demonstration of the error in application of the Doppler formula (1).
For our next experiment, we created waves with linear non-orthogonal wavefronts. The angle between the wavefront and the direction of wave propagation is no longer equal to 90 degrees.
Fig. 7 shows our new configuration. In the new configuration, the wavefront creates an angle between the wave propagation vector and the wave maximum line.
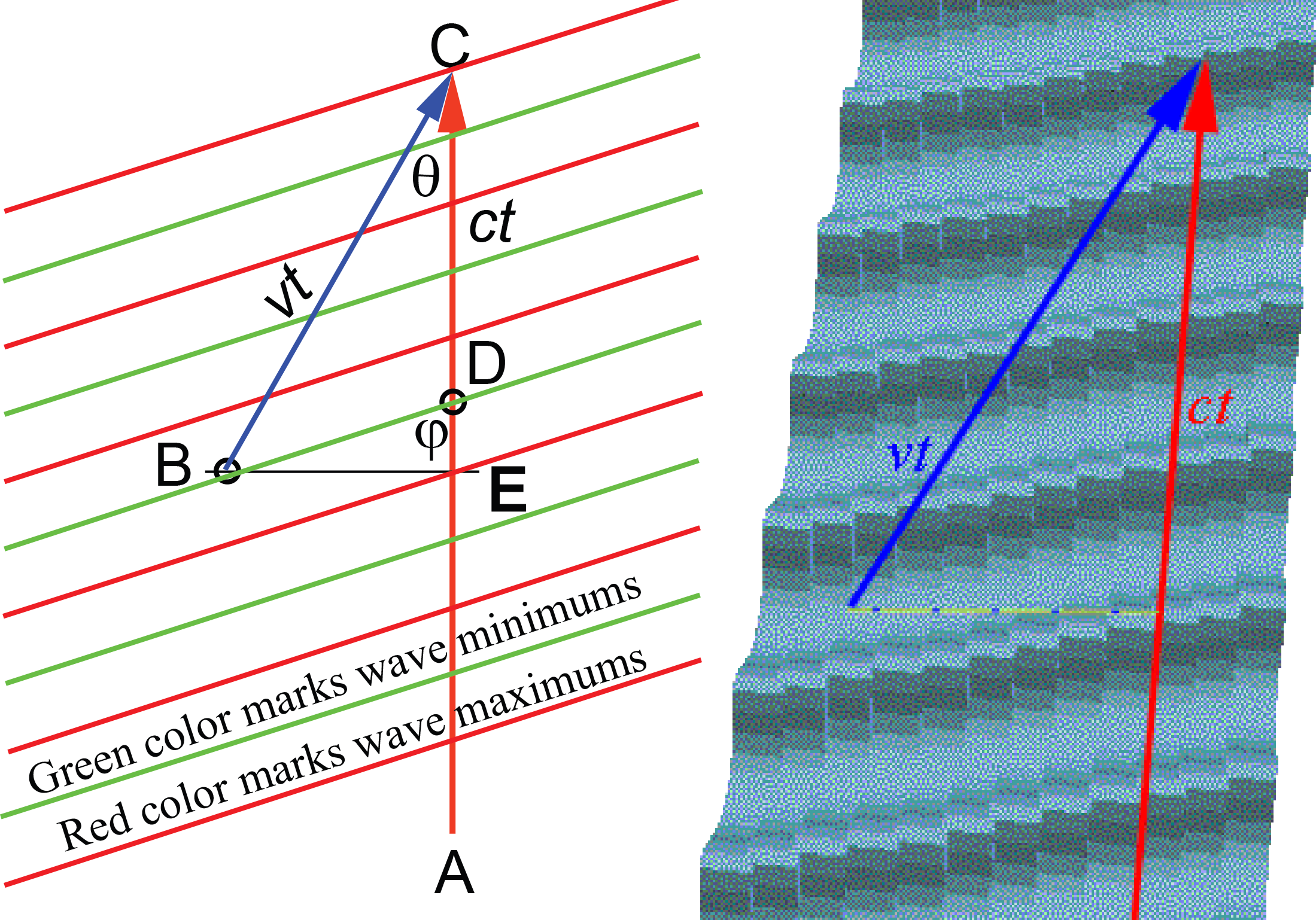
Fig. 7. Wave with linear non-orthogonal wavefront.
Point E denotes the position of the projection of the vector $v$ onto the direction of the vector $c$. Before the start of our experiment, when the initial time is zero, we note that two detectors at points B and E register a different phase of the wave – the minimum at point B and the maximum at point E. At the end of our experiment, both detectors arrive at point C simultaneously and register the same phase of the wave.
This means that two detectors that start moving simultaneously from points B and E and end moving simultaneously at point C, as a result, will calculate a different number of maxima. Therefore, at the end of the path, two detectors will show different values of the measured frequency. The phase shift between detectors B and E will constantly change from 180 degrees to zero during the time it takes to move both detectors to point C.
This is a demonstration of the case when the projection of the vector $v$ onto the direction of the vector $c$ gives an incorrect result.
The correct method uses the positions of the detectors on the line of the same phase of the wave.
To correct this error and restore the main idea of our method, we must place our detectors at points B and D along the line BD. This configuration restores the initial experimental conditions when each wave maximum (as well as the minimum or any line of the constant phase) intersects the position of detectors B and D simultaneously. This means that we satisfy all the conditions of the previous experimental part.
Instead of slit plates in this experiment, we use two motors at such a speed that both detectors reach point C at the same time.
To express our result in a mathematical formula, we must calculate the speed of the detector on the path DC and compare it with the speed of the detector along the line BC. Since the transit time is the same for both detectors, it will be quite sufficient if we compare the path lengths of both detectors. Obviously, the path length of one detector is BC = $v \cdot t$ . The path length of the other detector is DC and can be expressed as DC = EC – ED.
The length of the side EC = $vt \cdot cos \theta$. The length of ED = BE$ \cdot cotφ = vt \cdot sin \theta \cdot cotφ$.
In this expression, we used the angle $φ$ between the direction of wave velocity $c$ and the direction of the wavefront, i.e. line connecting the wave maxima (Fig. 7). This is the same angle as the angle between the line of wave fronts and the direction of wave propagation. The path length of the second detector can be found as DC = EC-ED:
DC = EC – ED = $vt \cdot cos \theta -vt \cdot sin \theta \cdot cotφ$ (20).
In order to calculate the wave frequency, the time during which we measure the wave maximums should be equal to 1 second.
We obtain the final expression for the general type of waves with a linear wavefront if we replace the collinear detector velocity $v$ in the Doppler formula (17) with our new expression for the detector speed obtained by dividing the distance DC from formula (20) by one second:
$f=f_0 [{1 \pm \frac{1}{c}( {v \cdot cos \theta { }- v \cdot sin \theta \cdot cot φ}})]$,
or
$f=f_0 [{1 \pm \frac{v}{c}( {cos \theta { } { } – sin \theta \cdot cot φ}})]$ (21).
The difference between our new formula (21) and the Doppler formula (18) is in parentheses of formula (21). Instead of $cos \theta$ we got an expression that depends on both the angle $\theta$ and the angle $φ$. If the angle $φ$ becomes equal to 90 degrees, then our formula (21) is transformed to the well-known Doppler formula (18) for waves with a linear orthogonal wavefront.
Our experiment proved once more that the formulation of the projection of the vector v onto the vector c as the basis for the formula (18) is incorrect. The idea of projection is misleading and gives the impression that it can be used for all kinds of waves.
Obviously, the idea of projecting the vector $v$ onto the vector $c$ should be replaced by an analysis of the lines and surfaces of the constant phase of the wave and accurate calculations of the number of wave periods per unit of time.
9. Waves with spherical and circular wave fronts.
The electromagnetic waves as well as acoustic waves with a spherical wavefront play a dominant role in modern technology. For example, radars or GPS devices use electromagnetic waves that have a spherical wavefront. If the area of the sonar emitter is small, compared with the area of the wavefront, then the acoustic waves will also have a spherical or circular wavefront.
The Doppler formula for both a spherical and a circular wave is incorrect, because the wavefront line CD does not coincide with the line CE which is the projection of the vector $v$ onto the direction of the vector $c$.
In addition, the angle between the vectors $v$ and $c$ is different for each point along the line CB. Any attempt to combine the wrong formula with the wrong angle will lead to a deliberately wrong result.
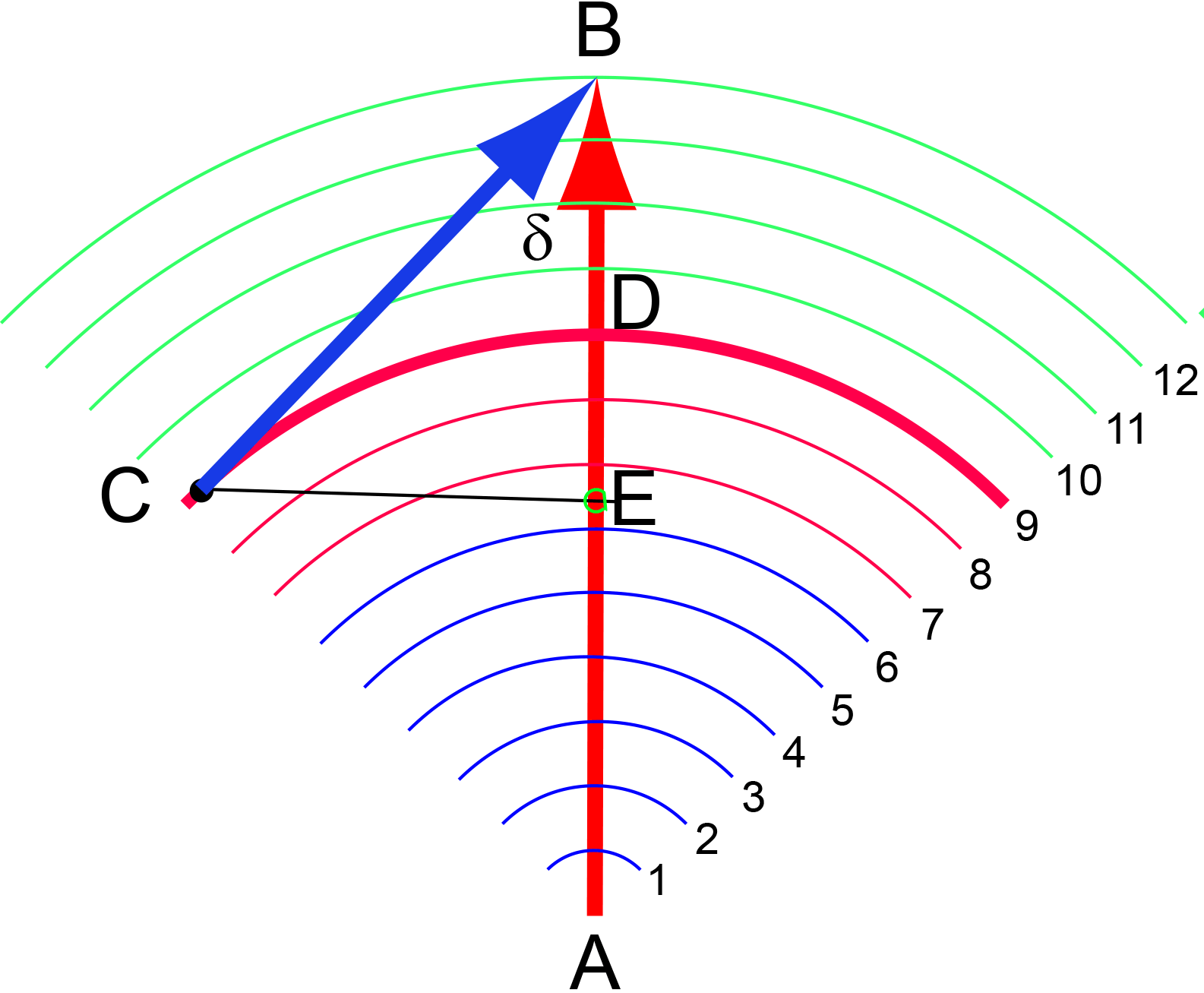
Fig. 8. A sector of the wave with circular wavefront.
For the Doppler Effect we have to analyze a section of the wave which propagates along the plane which contains both vectors $v$ and $c$. Our experimental method for waves with a linear wavefront can also be applied to waves with a spherical or circular wavefront. Fig. 8 shows a section of the cross section of a spherical wave and a plane with vectors $v$ and $c$. This is the part of a wave with a circular wave front.
Solid red lines in Fig. 8 indicate the positions of the wave maxima, and dotted green lines indicate the positions of the wave minima.
A small wave source is located at point A. A circular wave is emitted in all directions along the plane created by the vectors $v$ and $c$. One detector moves along the line AB collinear with the radius of the circles. It encounters another detector at point B.
Another detector moves from point C to point B. Its path is indicated by a blue arrow.
The aim of our method is to find the length of path of the first detector that moves along the red radial vector AC, provided that both detectors must register the same phase of the wave both at rest, before they begin to move to point C, and at any point during their movement to point C. If we fulfill this condition, both detectors will count the same number of waves during the same period of time.
The wave begins to move from point A and arrives at point B. The length of its path is AB = $c \cdot t$ . A non-collinear detector starts moving from point C and arrives at point B. The path length of this detector is CB = $v \cdot t$.
If we try to develop the idea of projecting the vector $v$ (blue arrow) onto the vector $c$ (red arrow), we notice that the detectors at points C and E at the beginning of our experiment will register a different phase of the wave. That would be a mistake.
According to our method, the initial positions of the two detectors should be at points C and D.
Before moving the detectors from their initial positions, we observe that each circular wave passes through both detectors C and D at the same time, and both detectors register the same number of waves.
Since the travel times for both detectors are the same, we can program two motors to move the detectors at such a speed that both of them start simultaneously from their respective positions at points C and D and simultaneously arrive at point B.
With this experimental configuration, the conditions of our method are satisfied, and we observe each wave maximum detected simultaneously by both detectors at all times.
In the analysis of this experiment we must express the result and conditions of this experiment in the form of a mathematical expression.
To obtain the formula for the experiment in Fig. 8, it is necessary to recall that the distance CB = $v \cdot t$, AB = $c \cdot t$. The distance AD is equal to the distance AC, because they are the radii of the same wavefront circle:
$AD=AC=\sqrt {c^2 t^2 + v^2 t^2-2\cdot v t \cdot c t \cdot cos\delta}$ (22).
The distance DB can be calculated as DB = AB-AD. Simple substitution of the values will result in:
$DB=ct – \sqrt {c^2 t^2 + v^2 t^2-2\cdot v t \cdot c t \cdot cos\delta}$ (23).
In the following expression, we put the factor ($c \cdot t$) out of brackets and get:
$DB=ct – ct ( \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})=ct(1 – \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})$ (24).
Since the frequency is calculated as the number of oscillations or wave periods in 1 second, we set the value of time t = 1. The speed of the collinear detector is equal to
$v=\frac{DB}{t} =c(1 – \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})$ (25).
Now we can replace the speed $v$ in the Doppler formula (17) with expression for speed (25):
$f = f_0[1-\frac {v}{c}]=f_0 [1 – \frac{c}{c} \cdot (1- \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})]$;
$f = f_0 \cdot ( \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})$ (26).
We replaced the plus / minus sign in equation (17) with the minus sign in equation (26), because there is no need to choose a sign for each direction of speed. (Note 1 below).
In the case of spherical waves, equation (26) is the correct formula for frequency conversion.
Notes:
1. We replaced the letter $\theta$ of an angle between the vectors $v$ and $c$ with the letter $\delta$. The angle $\theta$ in the Doppler formula (1.1) is limited to 90 degrees. But the angle $\delta$ in formula (3.5) has a range of plus/ minus 180 degrees as shown in Fig. 9.
In the case of non-inertial systems, such as circular orbit, the difference between the angles q and d will be observed even for angles less than 90 degrees.
Fig. 9. Illustration of the difference between the angles $\delta$ and $\theta$.
These angles will also be different in some other cases.
2. Instead of the plus / minus sign in the Doppler formula, we use only the minus sign. In the case of the opposite direction of the vectors $v$ and $c$, the mathematical expression of formula (26) will not change. The value of $cos \delta$ in this case will be negative, since the angle between the vectors $v$ and $c$ will be more than 90 degrees.
10. Error analysis.
In this section, we estimate the errors made in the case when the Doppler formula (1) was used to calculate changes in the frequency of waves with a spherical or circular wavefront.
How large is the improvement in accuracy in formula (26) compared with the Doppler formula (1) for spherical waves?
In the general case, we must compare these two methods for the case of objects that move in an arbitrary direction. For example, we look at an object that moves with speed $v$ at an angle $\theta=\delta$ relative to the radar beam.
The radar or sonar will measure the frequency of the emitted and reflected waves and calculate the speed in accordance with formulas (1) and (26). We will compare the difference in the results obtained using these two formulas and see if such an error should be neglected.
$f=f_0 ({1 \pm \frac{v { \ }cos \theta}{c}})$ (1)
$f = f_0 \cdot ( \sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta})$ (26).
In this example, the same radar or sonar measures for both methods the frequencies of the emitted $f_0$ and the observed $f$ waves. Thus, these values are the same for both methods. We divide both equations (1) and (26) by $f_0$.
The ratio ($\frac{f}{f_0}$) is the same in both formulas. This means that we can compare the right-hand sides of equations (1) and (26):
$\sqrt {1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot }cos\delta} = 1 – \frac{v cos \theta}{c} $ (27).
After squaring both the right and left sides of the equation, we get:
$1 + \frac{v^2}{c^2} – 2{\ } \frac{v}{c} { \cdot } cos\delta $ = $ 1 – 2 \frac{v}{c} cos \theta + \frac{v^2 cos^2 \theta}{c^2} $ (28).
For an inertial system and angles less than 90 degrees, the angles $\theta$ and $\delta$ are equal. The result is:
$v_{Durandin}=v_{Doppler} \cdot cos \theta$ (29).
The minus sign in the Doppler formula corresponds to angles less than 90 degrees. For angles greater than 90 degrees, the final result will be the same, because the sign in front of the third term on the left side of equation (28) and the sign in front of the second term on the right side of equation (28) will both change from negative to positive.
In the case when the angles $\theta$ and $\delta$ are equal to 60 degrees, the error in calculating the speed will be 100%, which means that the difference between the correct result and the result obtained by the Doppler method will be 2 times.
The smaller the value of $cos \theta$, the smaller the calculation error will be, and for the case of collinear motion, both methods will give the same result.
Reference.
Doppler, C. J. (1842). Ueber das farbige Licht der Doppelsterne und einiger anderer Gestirne des Himmels (About the coloured light of the binary stars and some other stars of the heavens). Publisher: Abhandlungen der Königl. Böhm. Gesellschaft der Wissenschaften (V. Folge, Bd. 2, S. 465-482) [Proceedings of the Royal Bohemian Society of Sciences (Part V, Vol 2)]; Prague: 1842 (Reissued 1903).