In 1905 Einstein published his paper “On the electrodynamics of moving bodies”.

This publication is known as Einstein’s Theory of Relativity. It is based on the results of Michelson’s experiments, in which Michelson tried to measure the speed of the Earth relative to the vacuum or ether. Einstein combined the description of Michelson’s experiment, the idea of Lorentz’s of two coordinate systems for two inertial systems and Poincare’s mathematical solution for electromagnetic waves transformations between two inertial systems. Einstein named that coefficient of transformations between inertial systems as the “relativistic beta”. These equations are known as the Lorentz transformations. Einstein also recognized the possible role of the Doppler Effect in Michelson’s experiment.
Although taken separately, Michelson’s experiment, the Lorentz transformations and the Doppler Effect all represent the correct parts of science, but their combination in the Theory of Relativity represents a bad combination.
Michelson’s experiments produced incorrect results because of a poor choice of light interference pattern shift, as a time and distance meter. Michelson also did not take into account the Doppler Effect.
The Doppler Effect could be able to help in the analysis of Michelson’s experiments, but Doppler’s formulas have a problem. They are correct only for linear waves, but Doppler’s formulas are incorrect for spherical electromagnetic waves.
In order to produce relativistic transformations of electromagnetic equations, Poincare used group symmetry of spherical waves.
As a result, Einstein’s Theory of Relativity consists of correct, but incompatible parts. In order to solve this problem it is necessary to find the general solutions for the Lorentz transformations and the general solutions of the Doppler Effect for spherical waves.
The corrections for the Doppler Effect formulas will be presented in Part 3 of this Project 1.
Here we present the general solution for the Lorentz Transformations.
Abstract:
1. We have corrected several errors in Einstein’s Special Relativity. The old expression for the relativistic β (Einstein):
$\beta=\frac{1}{\sqrt{1-{v^2\over c^2}}}$ (1),
which is correct for one point in space and time, we substituted with the new expression, which is correct for any point in space and time:
$\beta=\frac{1}{\sqrt{1+{v^2\over c^2} – 2{v\over c} cos{\delta}}}$ (2).
With the letter $\delta$ we marked the angle between vectors $v$ and $c$.
We agreed with H. Poincare’s statement that the Lorentz transformations can only be applied in the case of the geometry of the Michelson interferometer.
This makes the idea of the general applicability of Einstein’s Theory of Relativity, incorrect.
Our new expression (2) can be used for any point in space and time, because it represents the general solution of electromagnetic wave transformations for inertial systems.
Historical analysis.
The original or classic Theory of Relativity was formulated by Galileo. Newton later added his laws of Mechanics to Galileo Kinematics.
Now we call it the Galileo-Newton Theory of Relativity, and it states that the laws of Mechanics and Kinematics are invariant in any inertial system of coordinates.
It also means that no kinematics or mechanical experiment, made inside any closed inertial system, can help to determine the linear movement direction or speed relative to any other inertial system.
Classic Relativity cannot be applied to electromagnetic experiments, including optics.
In the 19th century, Michelson decided to measure the speed of the Earth relative to the vacuum (or relative to the Ether at that time). His experiments with the interferometer did not produce the expected outcome, and in the next hundred years, many scientists who conducted similar experiments have also not been able to achieve the expected outcome.
Based on these results Lorentz proposed the idea of extending relativistic properties to the electromagnetic field. He introduced relative coordinates and relative time for any inertial system:
$x=x_i \pm vt$, $t=t_i \pm \frac{xv}{c^2}$ (3).
Poincare indicated that these coordinates cannot solve the problem with the results of Michelson’s experiment, because the Maxwell equations of the electromagnetic field would still lose their spherical symmetry and change their form as a result of the transition between the different inertial systems.
Poincare introduced his normalization coefficient.
He also underlined that these transformations are not valid in general application, and would be correct only for the geometry of Michelson’s interferometer experiment.
Einstein ignored the advise of Poincare and claimed that the Lorentz transformations can be applied universally.
We shall analyze both Poincare’s and Einstein’s points of view, and explain which of these scientists made a mistake.
Einstein’s publication “On the electrodynamics of moving bodies” has two main parts: “Kinematical Part” and “Electrodynamical Part”.
The paragraph about the Relativistic Doppler Effect we put in a separate part, because of its importance for this theory.
Part 1. “Kinematical Part”.
We shall start our analysis from the first part, which is called “Kinematical Part”. The first and second paragraphs of Einstein’s publication introduce the definition of simultaneity, time interval, the constant character of the speed of light, etc.
The Theory of the Time and Space Transformations starts in the third paragraph.
In the description of Einstein’s imaginary experiment, the solid rod with the mirror at the end is just a simplified description of the Michelson interferometer. The first equation describes the travel of the light in the longitudinal shoulder of the Michelson interferometer. Here Einstein made his first error, when he claimed that the distance $x’$ is infinitely small and substituted it with the differential $dx$. Since both the speed of light and the speed difference of the inertial systems are constant, there is no need for this differential. Also – by making this substitution – Einstein restricted his theory to the cases of infinitely small speeds $v$ of inertial systems. When applied in a general case, the speed of the inertial system cannot be neglected and this substitution becomes a rather significant error.
Einstein introduced the most important transformation on the same page without any explanations. Instead of carefully analyzing the propagation of light in the transverse shoulder of the interferometer, Einstein simply states: ”…being borne in mind that light is always propagated along these axes, when viewed from the stationary system, with the velocity
$\sqrt{c^2-v^2}$ ” (4).
Einstein did not explain the logic behind this normalization parameter. In his imaginary experiment there is no need for a perpendicular shoulder of Michelson’s interferometer. He was supposed to compare the case of the moving rod with the ideal case of the rod at rest, when the speed of the inertial system is equal to zero.
It is easy to notice that the comparison of the distance in the first inertial system ($D=c \cdot t$) and in the second inertial system, calculated from equation (4) result in the equation:
$c \cdot t_1 = \sqrt{c^2 – v^2} \cdot t_2$ (5).
There is no need for the rest of the calculations. If we divide both sides by the speed of light $c$, the result will be relativistic $\beta$.
This normalization parameter (4) was already introduced and explained by Poincare. Einstein simply borrowed this parameter and combined it with Lorentz’s relative coordinates. As a result, Einstein produced equations, currently known as the Lorentz transformations:
$x = \frac{x_i -vt}{\sqrt{1-{v^2 \over c^2}}}$, $y = y_i$, $z = z_i$,$t = \frac{t_i – \frac{xv}{t^2}}{\sqrt{1-{v^2 \over c^2}}}$ (6).
Einstein here introduced the parameter beta:
$\beta = \frac{1}{\sqrt{1-{v^2 \over c^2}}}$ (1).
Without relativistic $\beta$, these expressions are simply Lorentz’s relative coordinates and relative time:
$x = x_i -vt$ and $t = t_i – \frac{xv}{t^2}$ (3).
Unfortunately, when these expressions are combined with the relativistic $\beta$, it is necessary to notice that Lorentz’s relative coordinates are correct for any point in space and time, but Einstein’s relativistic beta is correct only for the case, when the points of origin of both relativistic inertial systems coincide at the time with the start of the measurement. It means that in these equations, initial values of $t$ and $x$ must be equal to zero, because for any other values of $t$ or $x$ the relativistic $\beta$ is not correct.
The argument about moving the coordinate system cannot be accepted seriously. It is impossible to move a telescope to another planet. And where do you put your coordinate system origin if you need to observe two objects, moving in opposite directions.
In order to find the GENERAL SOLUTION (general relativistic $\beta$) of the Maxwell equations for two inertial systems we used two approaches. In the first approach we used a polarized coherent train of probe photons which propagated in the x-direction with their electric field restricted in $x-y$ plane and magnetic field restricted to $x-z$ plane. We took into account that the energy of both electric and magnetic field over any one period of wave is equal to photon energy $E=hf$ .
As the result of our calculations, we got the expression for the general relativistic beta as:
$\beta=\frac{1}{\sqrt{1+{v^2\over c^2} – 2{v\over c} cos{\delta}}}$ (2).
The second approach is similar to Poincare’s method, which uses group symmetry. In the case of Maxwell’s equations, this solution is simplified by the fact that the transformation for the spherically symmetrical group is equivalent to the transformation of the point of origin. The result is the same formula.
Einstein’s original publication has no illustrations, but modern books about relativity have them. Usually the first illustration looks similar to Figure 1:
Figure 1. Two inertial systems. At time zero second system starts to move with the speed v relative to the first system in the direction parallel to the x axis.
The points of origin of these two coordinate systems are marked with the letters O and Oi. When the time is equal to zero, both points of origin are located at the same point in space. At the start of the chronometer, one of the systems starts moving along the $x$– axis with a constant speed $v$. These conditions correspond to the imaginary experiment described by Einstein, as well as for the Michelson’s interferometric experiment. According to Figure 1
$cos {\delta} = \frac {v}{c}$.
If we substitute this condition into our general formula (4), we shall get Einstein’s expression for $\beta$:
$\beta=\frac{1}{\sqrt{1+{v^2\over c^2} – 2{v\over c} cos{\delta}}}$ $=\frac{1}{\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{v\over c}}} $ $=\frac{1}{\sqrt{1-{v^2\over c^2}}}$ (7).
This is the proof that Einstein’s $\beta$ is just one part of our general solution. It is also a proof that Einstein’s $\beta$ is correct only for one specific point in space, when the points of origin of both inertial system coincide, vector $v$ is parallel to $x$-axis and ${v_y} = {v_z} = 0$.
The argument that the point of origin can be moved arbitrarily is wrong from a practical point of view. As an illustration, let us take a look at Figure 2 and Figure 3:
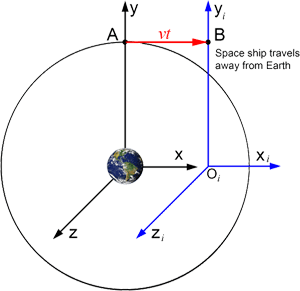 Figure 2.
Figure 2. 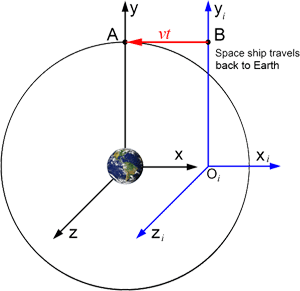 Figure 3.
Figure 3.
Figure 2 and Figure 3 are similar to Figure 1. We added the Earths at the origins of coordinates and indicated the orbit of spacecrafts as black circles.
Figure 2 represent the case, when spacecraft occupy the orbit of Earth before it starts a trip to another planet along the line AB.
Figure 3 represents the case when spacecraft returns from travel, to the orbit of Earth also along the line AB.
Figure 3 is an example of the case, where Lorentz transformations and Einstein’s beta are not correct. In the case of Figure 3 it would be difficult to move Earth, or even only a NASA headquarters with all of their equipment to the point outside the Earth fast enough in order to produce any measurement.
Even in case of Figure 2, only one first measurement can use Einstein $\beta$. For any next point, which is not on the $Y$ axis that $\beta$ is incorrect.
And try to imagine where you would put coordinates origin, when two spaceships simultaneously approach you from two different sides. (We shall discuss it later, not in this project, when we start discussions about $E=mc^2$.)
For the specific combination of the spacecraft speed and position on Figure 3, the formulas for the relativistic transformations can be written as:
$x = \frac{x_i + vt}{\sqrt{1+{v^2 \over c^2}}}$, $y = y_i$, $z = z_i$, $t = \frac{t_i + \frac{xv}{t^2}}{\sqrt{1+{v^2 \over c^2}}}$ (8).
This result can be obtained if you put $cos \delta = 0$ into our new formula for general relativistic $\beta$.
Both these transformations and the original Lorentz transformations have no practical meaning, because they represent not the general solution, but only another specific solution for the invariant of the Maxwell equations of the electromagnetic field, which is valid only for one point in space and time. These formulas also prove that Poincare was right, when he wrote that the Lorentz equations can only be used for the geometry of the Michelson interferometer.
For any initial value of $x$, other than $x = 0$ the relativistic
$\beta=\frac{1}{\sqrt{1-{v^2\over c^2}}}$ (1).
would be incorrect.
Since publication of these space and time transformations with the plus sign instead of minus, they became one of the major points of confusion.
Obviously, these formulas are not compatible with the Minkowski equation, as well as with some theorems for Minkowski space.
It is an obvious mistake in the Theory of Special Relativity and we shall analyze this mistake now.
1. The Minkowski equation is based on Poincare’s four-dimensional space representation (x,y,z,t).
2. Unfortunately Minkowski followed Einstein into the same mistake by ignoring Poincare’s statement that such space as well as the relativistic $\beta$, and the Lorentz transformations, are restricted by the geometry of Michelson’s experiment. For the Minkowski equation, the distance between the points of origin of the two coordinates systems must increase as a function of time. If the distance between the origins of two inertial systems decreases, then Minkowski equation become incorrect.
3. For the further analysis of the Minkovsky equation please do not be confused by circles on Figure 2 and Figure 3. The circles on Figures 2and 3 represent the ORBIT of the spaceship around the Earth, but NOT the SPHERE OF ELECTROMAGNETIC WAVES. Figures 2 and 3 were created in order to show the practical case, where the solution for both movement toward and away from the coordinate origin are important for modern technology.
4. For Minkowski case Figures 4 and 5 below show the case, where the circles represent the spheres of the electromagnetic waves, which start at the point of origin and reach our moving object at point C. Object moves away from point of origin along line AC (Figure 4). In the other case the object start from arbitrary point B and moves along the line BC (Figure 5) toward the origin of the coordinate system, until it meets electromagnetic wave, emitted from the point of coordinates origin.
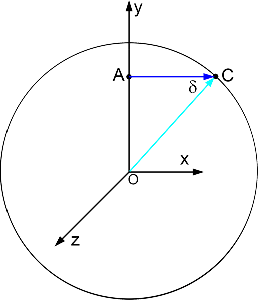 Figure 4.
Figure 4. 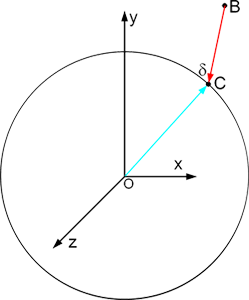 Figure 5.
Figure 5.
It is easy to notice that in the first case, when the distance between the object and the source of the wave increases (Figure 4) the point, from which the object started, as well as every point along the line AC are located inside the sphere of the wave. Point A can occupy any position, not necessary on coordinate axis.
In the opposite case, when the distance between the object and the source of the wave decreases (Figure 5), the initial point of the object and any point on the line BC are located outside of the circle of the wave.
The Minkowski equation can be written as
$x^2 + y^2 + z^2 = c^2t^2$ (9).
For any moment of time the radius of the sphere of wavefront is equal to $ct$. Coordinates $x, y, z$ – represent coordinates of positions of the moving object. For object, which moves away from the origin of coordinates, the sum of the second powers of coordinates is equal to the second power of the radius $ct$ of the wave sphere. Minkowsky equation is correct for such object. This is the case for Figure 4.
But Minkowsky equation is obviously not correct for Figure 5. The value of the $y$-coordinate of the point B is bigger, than the radius of the sphere. The second power of this one value of coordinate $y$ is already bigger than the radius $ct$. The same is correct for any point along the line BC on Figure 5.
It means that the Minkowski equations cannot be used for any inertial system, when the distance between these systems decrease, as is the case for a spaceship, returning home.
The Durandin invariant has a general character and the Minkowski equation can be considered as a part of the Durandin solution for the case, when the angle $\delta < 90$ degrees. It is easy to check that for all points inside the sphere of the wave, the Durandin solution will produce the same results as the solution of the Minkowski equation in that case.
We shall skip analysis of paragraphs 4 and 5 of Einstein’s publication, because they are not important for this project. We will return to them, if it will be necessary.
Part 2. “Electrodynamical Part”.
Paragraph 6 of the Part 2 of Einstein’s publication describes the transformation of the electromagnetic field in inertial systems. Taking into account that the goal of the Theory of Relativity is invariant of the electromagnetic field, this part of Einstein’s paper is the most interesting and also the most contradictive.
“These Maxwell-Hertz equations of the electromagnetic field
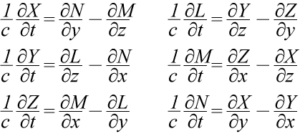
where (X, Y, Z) denote the vector of the electric force, and (L, M, N) that of the magnetic force, describe spherical wave in the coordinate system at rest” (Einstein).
Einstein’s goal in this section was to show that these equations of the electromagnetic field will be invariant in the second set of coordinates, associated with the moving inertial system. It would be logical to write two systems of equations for two inertial systems and try to find the value of parameters, such that equations are invariant.
However Einstein’s approach to this problem is rather surprising.
First he applied the Lorentz transformation (from Part I) to this set of equations:
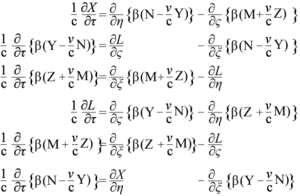
The next step is quite confusing. Instead of solving these sets of equations, Einstein wrote:
“If we now form the reciprocal of this system of equations, firstly by solving the equations just obtained, and secondly by applying the equations to the inverse transformation (from k to K), which is characterized by the velocity -v, it follows, when we consider that the two systems of equations thus obtained must be identical, that “.
Whatever was the goal of these forward-backward coordinate transformations, it is clear that Einstein did not find any solution to the Maxwell equations of the electromagnetic waves. He simple supposed that Lorentz transformations represent the general solution.
At the end of this paragraph Einstein speculated about the “electromotive” and “magnetomotive” forces, which act upon the probe charge. For this part we should excuse Einstein, because in 1905 he could not know about the quantum character of the interaction between the particles (or dipoles) and electromagnetic waves.
But we have to make a note about the Einstein’s approach to proving this idea.
Forward – backward transformations, as well as general look of equations prove nothing.
As an example let us write the equation:
$Length [A(0,4) + B(3,0)] = Length [C(x,y)] $, where A, B, C are vectors.
We can easy guess that vector C(3,4) can satisfy this equation. If we use the same approach as Einstein, we first transform vectors A, B and C into polar form
$Length [A(R_1,φ_1) + B(R_2,φ_2)] = Length [A(R_3,φ_3)]$.
After that we shall make inverse transformations and get our initial equation. These transformations have nothing to do with the general solution of the problem.
Although we were able to guess the correct value of the vector, which satisfies our equation, the general solution for this problem is the circle with the radius $R=5$.
The specific invariant, found by Poincare and Einstein, is correct for one specific case, but it is not the only possible specific solution. There are several other specific solutions. Each of them is not correct in the general case, but need some specific conditions in order to be correct.
For example, in order to apply Lorentz transformations it is necessary that initial values of
$x = 0, y ≠0, V(x) ≠0, V(y) = 0$ (10).
If any one of these conditions is changed, then the Lorentz transformations would become invalid.
We have to remember that some aspects of the modern physics were not known at the beginning of the 20th century. In our present day, it is possible to use an approach, where the electromagnetic wave is considered as an ensemble of single photons. We can choose such group of photons, which create a coherent polarized wave.
The general solution in this case can be found by reducing the rank of the tensors and rotating the coordinate axes around the point of origin until the vectors create a plane such that the direction of the wave propagation coincide with the x– axis, the electric field changes along the z – axis and the magnetic field changes along the y – axis. This procedure is adequate, because for this type of group transformation, as well as for the (general) relativistic invariant the result of axes rotation around the origin of coordinates is equal to one.
In the absence of distortions it is possible to use the energy symmetry of the electric and magnetic fields. (This solution was the first that we found. Later it became clear that the approach, where spherical symmetry of the wave is combined with the rotation and shift of the coordinate axes, similar to the one used by Poincare, gives the same result without any need of matrix manipulation). The integrals of electric and magnetic components of electromagnetic wave over time, equal to one wave period, are equal. This results in the wave transformations equal to transformations of position of points of waves origins.
The solution represents the general invariant of the Maxwell equations:
$\beta=\frac{1}{\sqrt{1+{v^2\over c^2} – 2{v\over c} cos{\delta}}}$ (2).
Here $\delta$ is the angle between the vectors $v$ and $c$. It is easy to show (see in part 3, below) that this solution includes both Lorentz transformations and all other specific cases.
The new general form of the Lorentz transformations will be:
$t_i =\frac{{t \pm \frac{A(x,y,z) V(v_x,v_y,v_z)}{c^2}}} {\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{cos\delta}}}$ $B_i (x_i, y_i, z_i) =\frac{{A(x,y,z) \pm V(v_x,v_y,v_z) t}} {\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{cos\delta}}}$ (11).
If we substitute separate projections of the speed with vector $V(x, y, z)$, then the plus/minus sign can be substituted with just the minus sign, provided we can agree that the speed vector has a positive sign, when the object moves away from the origin of the coordinates, and the speed vector becomes negative, when the distance between our object and the origin of the coordinates system decreases.
NOTE: These formal mathematical transformations are not an indication that we agree with the general idea of such space and time transformations. Quite the opposite. The physical meaning of these transformations were discussed in the first part and will be further discussed in another lecture. We proved that scientific time and space are linear and uniform. All non linearities are the result of inappropriate use of instrumentation , such as clocks and meters during experiments and in theoretical models.
Part 3. Relativistic Beta: General Invariant vs Specific Solutions.
Now let us take a look at some of the specific solutions, which can be obtained by applying proper conditions to our general solution for relativistic beta:
$\beta=\frac{1}{\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{cos\delta}}}$ (2).
1. In the case of Einstein’s Theory of Relativity, the Poincare invariant (as was stated by Poincare) is valid only for the geometry of the Michelson experiment. The initial condition for this case can be formulated as, (see Figure 1):
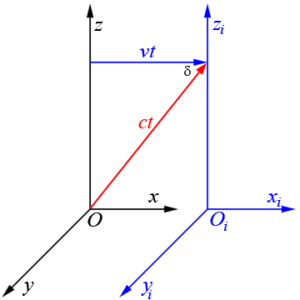 Figure 1.
Figure 1.$cos \delta = {v \over c}$ (12).
Substitution of this expression (Figure 1) into the Durandin general invariant gives us Einstein’s Relativistic invariant:
$\beta=\frac{1}{\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{v\over c}}}=\frac{1}{\sqrt{1 -{ v^2\over c^2}}}$ (13).
2. The case, corresponding to Figure 3 where the spaceship is returning back to Earth’s orbit, has the condition $cos\delta = cos 90 = 0$.
 Figure 3.
Figure 3.
$\beta=\frac{1}{\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{cos 90}}}=\frac{1}{\sqrt{1 +{ v^2\over c^2}}}$ (14).
This result is correct only at the coordinate point, which is very close to the y– axis and only for the direction, which is perpendicular to the y– axis.
3. In the case of a satellite, moving in a perfect circular orbit (Figure 6 below) the $cos \delta = {v \over 2c}$:
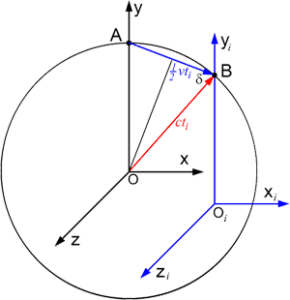 Figure 6.
Figure 6.
When we substitute this expression for angle $\delta$ the general invariant becomes:
$\beta=\frac{1}{\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{v\over 2c}}}=1$ (15).
This is a trivial result, because this result $(\beta = 1)$ for circular movement can be obtained from the spherical symmetry of group transformations. It can also be obtained by the rotation of the coordinate axes around their point of origin. Such transformations are equivalent to the rotation around the point of coordinate origin. So the idea of time correction for the satellites, rotating around the Earth, is totally wrong. Obviously the idea to grab the clock and travel around the Earth cannot be used to check Einstein’s theory. It is nonsense.
There is no doubt that other special cases can be formulated and solved, if it would be necessary.
Regarding spherical transformations, practically, it is also impossible to put a NASA control center at the center of the Earth. Also the orbit of satellites is almost never a perfect circle, which means that it is still another impractical specific case.
The next important part in Einstein’s publication is:
Part 4. Relativistic Doppler Effect.
Einstein’s first formula in Part 4 is:
$\nu’=\nu \frac{1 – \frac{v { \ }cos φ}{c}}{\sqrt{1 -{ v^2\over c^2}}}$ (16).
where $\nu’$ and $\nu$ – are the wave frequencies for the moving inertial system and the system at rest.
It is easy to see that Einstein substituted plus/minus ($\pm$) sign in the Doppler formula with just minus ($-$) and then simply multiplied the Doppler formula:
$\nu’=\nu {(1 – \frac{v { \ }cos φ}{c})}$ (17)
by his relativistic beta:
$\beta=\frac{1}{\sqrt{1-{v^2\over c^2}}}$ (1).
It is a clear mistake and not just a single mistake, but several mistakes. Let us take a closer look.
- This is a wrong type of the relativistic $\beta$, because this formula for $\beta$ is not general and it is not correct for an arbitrary position, speed or direction. Doppler’s formula (with plus/minus ($\pm$) sign) can be used for any direction.
- The Doppler formula cannot be used for the spherical electromagnetic waves. (We shall discuss Doppler Effect and this problem in more details in our next Part 3). The original Doppler formula is correct only for the linear type of orthogonal waves. (There is one special case of the collinear orthogonal vectors, when the Doppler formula coincides with the correct formula for spherical waves and it can give an accurate result).
- There is no scientific basis for multiplying an arbitrary parameter by the relativistic $\beta$. Relativistic $\beta$ is not an abstraction. It is part of the formulas and, according to Einstein, it can be used only in the time or space transformations. In science, such multiplication leads to new measurement units. In this case, if Einstein multiplied the relativistic distance (space) by Doppler’s frequency, the result will not be a frequency but a speed, and the units will be:
$length { \ }(m) { \cdot } frequency{ \ }({1\over {sec}}) = speed{ \ } ({m \over {sec}})$ (18).
If instead of length Einstein decided to multiply relativistic time by Doppler’s frequency, the result would be not a frequency, but an abstract formula without units:
$time{ \ }({sec}) { \cdot } frequency { \ }({1\over{sec}}) = ???{ \ } ({sec \over {sec}})$ (19).
Maybe somebody believes that Einstein did not know that units of measurements are transformed according to the formulas?
We shall leave this question without answer.
It is obvious from points 2 and 3 above that the relativistic Doppler formula cannot be used for the frequency changes calculations in the case of electromagnetic waves and should instead be substituted with the Durandin formula for spherical wave frequency transformations:
$f = f_0 { \ }{\sqrt{1 +{ v^2\over c^2} -2 {v\over c}{cos\delta}}}$ (20).
(We shall produce and discuss in details this formula in the Part 3 of Project 1, named “The limits of applicability of the Doppler Theory”.)
In this formula $f$– and $f_0$ – are measured and emitted frequencies, $v$– is the speed of the object, $c$– is the speed of the electromagnetic wave and $\delta$– is the angle between these speeds. But this angle is not always the same, as the angle $\theta$, which is used in the Doppler formula.
The first difference is the range of change. The angle $\delta$ in the Durandin formula has range of values between $0$ degrees and $180$ degrees. The angle $\theta$ in the Doppler formula can have values between $0$ and $90$ degrees. Other differences will become obvious, when the Durandin method will be applied to non-linear movement, such as the orbital movement of the satellites or for the time synchronization between the Earth command center and satellites or planes. (More details about this formula in the Part 3 of Project 1).
At the end of the 7th paragraph, Einstein forgot that his Theory of Relativity was created for the case, when vectors $v$ and $c$ are parallel, but not collinear, as well as about the condition that the direction of the vectors $v$ and $c$ cannot be opposite.
Einstein came to the wrong conclusion that the frequency in such a case would be: “…when $v = -c,{ \ } \nu = \infty$ .
This statement contradicts the Law of Energy Conservation.
If Einstein were correct, it would be enough to accelerate one molecule to the speed close to the speed of light and illuminate it with a single photon. One photon, absorbed by an accelerated molecule would be enough to produce an infinite amount of energy according to Planck formula $E = h{ \cdot }f$.
Unfortunately it is simply another mistake and the correct result will be $\nu’ = 2{ \ }\nu$ .
This result means that for astronauts the red stars will look like ultraviolet stars, if their spaceship moves with the speed close to the speed of the light.
Later in this paper Einstein introduced the idea of the longitudinal and transverse masses:
$Longitudinal{ \ } mass =\frac{m}{({ \ }\sqrt{1 -{ v^2\over c^2}}{ \ })^3};{ \ }Transverse{ \ } mass =\frac{m}{{ \ }{1 -{ v^2\over c^2}}{ \ }}$ (21).
These formulas are obvious mistake. They even contradict to Einstein’s formula $E=m{ \ }c^2$. Internal energy of the particle cannot have two different values at the same time.
The rest of this paper is also a mistake.
There is no need to say that the idea of the wormholes, different kind of singularities, as well as several other parts of science, associated with Einstein’s Relativity need corrections.
The next Part of this Project is “The limits of applicability of the Doppler Theory”.



 Figure 2.
Figure 2.  Figure 3.
Figure 3. Figure 4.
Figure 4.  Figure 5.
Figure 5.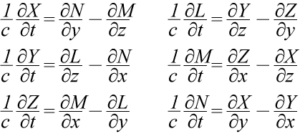
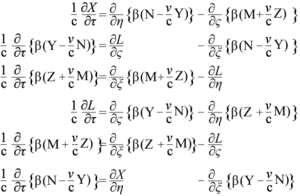
 Figure 1.
Figure 1. Figure 3.
Figure 3. Figure 6.
Figure 6.